
Bond order of
A. 1
B. 2
C. 3
D. 0
Answer
493.2k+ views
Hint: According to the molecular orbital theory, the bond order is defined as the number of covalent bonds in a molecule. Bond order is equal to half of the difference between the number of electrons in bonding (
Complete Solution :
A Be atom has four electrons. It has two valence electrons and its electronic configuration is
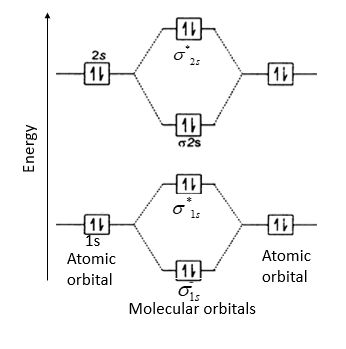
Thus, electronic configuration of
Here, bonding electrons,
Therefore, bond order (B.O.) of
- Zero value of bond order corresponds to non-existence of
So, the correct answer is “Option D”.
Note: The bond order of a molecule conveys the following information:
1. The stability of a molecule can also be expressed in terms of bond order. Higher the bond order, more stable is the molecule.
2. Bond length: Bond order and bond length are inversely related. Thus, higher the bond order, shorter is the bond length and vice-versa.
3. Bond dissociation energy: Bond order in a molecule is directly proportional to its bond dissociation energy. Greater the bond order, more will be the value of bond dissociation energy.
Complete Solution :
A Be atom has four electrons. It has two valence electrons and its electronic configuration is

Thus, electronic configuration of
Here, bonding electrons,
Therefore, bond order (B.O.) of
- Zero value of bond order corresponds to non-existence of
So, the correct answer is “Option D”.
Note: The bond order of a molecule conveys the following information:
1. The stability of a molecule can also be expressed in terms of bond order. Higher the bond order, more stable is the molecule.
2. Bond length: Bond order and bond length are inversely related. Thus, higher the bond order, shorter is the bond length and vice-versa.
3. Bond dissociation energy: Bond order in a molecule is directly proportional to its bond dissociation energy. Greater the bond order, more will be the value of bond dissociation energy.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE




