
Between the plates of a parallel plate capacitor a dielectric plate is introduced just to fill the space between the plates. The capacitor is charged and later disconnected from the battery. The dielectric plate is slowly drawn out of the capacitor parallel to the plates. The plot of the potential difference across the plates and the length of the dielectric plate drawn out is:
Answer
412.8k+ views
Hint: When a parallel plate capacitor is filled up with a dielectric material, its capacitance will be increased by
The voltage drop across the capacitor is inversely proportional to its capacitance. If capacitance increases voltage drops across the capacitor decreases and vice versa.
Complete step by step solution:
When a parallel plate capacitor is filled with free space, its capacitance
Where,
Let
When the capacitor is filled with dielectric material having the dielectric constant
Now the capacitor filled with dielectric is charged and then disconnected from the battery. The dielectric material is slowly drawn out of the capacitor parallel to the plates.
We can consider this situation as two capacitors
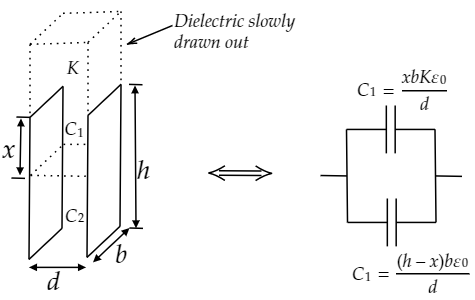
Let
Then the height of the parallel plates of the capacitor
Surface area of a parallel plate of
The capacitance
In the same way,
And
The total capacitance of the two capacitors connected in parallel is
Substitute the values of
Further simplifying
We know that the voltage drops
Voltage drops
Substitute the value of
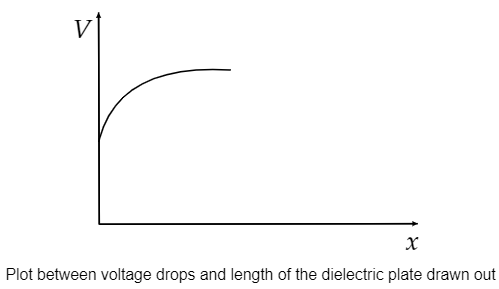
So, when the dielectric material is slowly drawn out from the capacitor,
So, the graph between voltage drops and length of the dielectric plate drawn out is as follows.
Note:
It should be noted that the total charge of the capacitor remains constant. When the two capacitors are connected in parallel, its effective capacitance will be the sum of capacitance of each capacitor.
The dielectric constant for a given material is the ratio of permittivity of the material to the permittivity of free space. The dielectric constant is also known as relative permittivity.
The voltage drop across the capacitor is inversely proportional to its capacitance. If capacitance increases voltage drops across the capacitor decreases and vice versa.
Complete step by step solution:
When a parallel plate capacitor is filled with free space, its capacitance
Where,
Let
When the capacitor is filled with dielectric material having the dielectric constant
Now the capacitor filled with dielectric is charged and then disconnected from the battery. The dielectric material is slowly drawn out of the capacitor parallel to the plates.
We can consider this situation as two capacitors

Let
Then the height of the parallel plates of the capacitor
Surface area of a parallel plate of
The capacitance
In the same way,
And
The total capacitance of the two capacitors connected in parallel is
Substitute the values of
Further simplifying
We know that the voltage drops
Voltage drops
Substitute the value of
So, when the dielectric material is slowly drawn out from the capacitor,
So, the graph between voltage drops and length of the dielectric plate drawn out is as follows.

Note:
It should be noted that the total charge of the capacitor remains constant. When the two capacitors are connected in parallel, its effective capacitance will be the sum of capacitance of each capacitor.
The dielectric constant for a given material is the ratio of permittivity of the material to the permittivity of free space. The dielectric constant is also known as relative permittivity.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is insulin not administered orally to a diabetic class 12 biology CBSE

How do you convert from joules to electron volts class 12 physics CBSE

Why should a magnesium ribbon be cleaned before burning class 12 chemistry CBSE

The first microscope was invented by A Leeuwenhoek class 12 biology CBSE

The current flowing through the resistor in a series class 12 physics CBSE




