
Assuming mass m of the charged particle, find the time period of oscillation.

Answer
507.3k+ views
Hint: The acceleration of a charge is equal to the product of square of angular frequency and the displacement. The force exerted on the charge is equal to the product of charge and the net electric field. By equating it with Newton’s second law, we can get acceleration and from acceleration we can get angular frequency and finally the time period.
Formula used:
Newton’s second law of motion:
Force on a charge in electric field:
Acceleration of an oscillating charge is given as
Time period is related to angular frequency by following relation:
Here all the symbols have their usual meaning.
Complete answer:
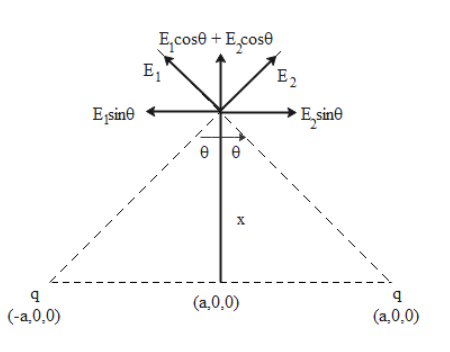
In the given diagram, we have two charges of the same magnitude at (-a,0,0) and (a,0,0). At a point along the line passing through the centre of the line joining the two charges, the various components of the electric field are shown. Since the charges have the same magnitude their electric fields also have the same values.
Now the horizontal components of the electric field cancel each other while the vertical components get added up. Hence, the net electric field is given as
The force due to this electric field on a charged particle
If x << a then,
Now using Newton’s second law, we get
We denote acceleration by A.
As we know that acceleration of a charged particle undergoing oscillations is given as follows:
The time period is given as
Hence, the correct answer is option B.
Note:
In this question the charge that is undergoing oscillation is a positive test charge. But in case, we had a negative charge then the directions of resultant forces would have been opposite to that for the positive charge. But the final answer would still remain the same in magnitude.
Formula used:
Newton’s second law of motion:
Force on a charge in electric field:
Acceleration of an oscillating charge is given as
Time period is related to angular frequency by following relation:
Here all the symbols have their usual meaning.
Complete answer:
In the given diagram, we have two charges of the same magnitude at (-a,0,0) and (a,0,0). At a point along the line passing through the centre of the line joining the two charges, the various components of the electric field are shown. Since the charges have the same magnitude their electric fields also have the same values.
Now the horizontal components of the electric field cancel each other while the vertical components get added up. Hence, the net electric field is given as
The force due to this electric field on a charged particle
If x << a then,
Now using Newton’s second law, we get
We denote acceleration by A.
As we know that acceleration of a charged particle undergoing oscillations is given as follows:
The time period is given as
Hence, the correct answer is option B.
Note:
In this question the charge that is undergoing oscillation is a positive test charge. But in case, we had a negative charge then the directions of resultant forces would have been opposite to that for the positive charge. But the final answer would still remain the same in magnitude.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




