
Assuming 2s-2p mixing is not operative the paramagnetic species among the following is:
A)
B)
C)
D)
Answer
495.6k+ views
Hint: The magnetic property of a molecule can be explained based on the molecular orbital theory. The molecule which does not contain the unpaired electron is known as the paramagnetic. The molecule which has all-electron paid-up does not contribute towards the magnetic property. It is diamagnetic in nature. To solve such a problem write down the MOT diagram of molecules.
Complete step by step solution:
The MOT diagram is drawn in such a way that we do not consider the s and p orbital mixing. Therefore the order of orbitals is not changed.
Let’s first draw the MOT of all molecules given in the problem.
A)
The
The MOT is as shown below,
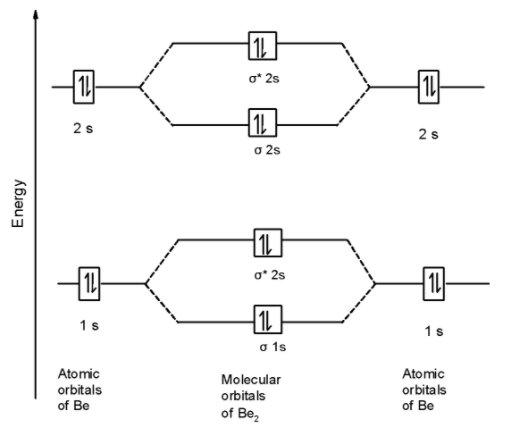
First of all, we can write the molecular orbital configuration of
Since, here all molecular orbitals contain the two electrons. These are all paired electrons. Thus
B)
The
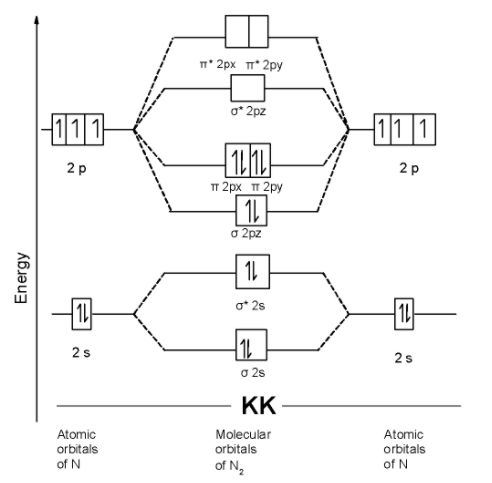
We can write the molecular orbital configuration of
Since, here all molecular orbitals contain the two electrons. These are all paired electrons. Thus
C)
The
The MOT is as shown below,
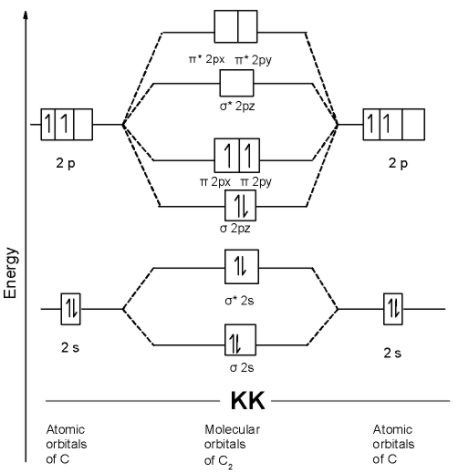
we can write the molecular orbital configuration of
Since the pi molecular orbitals contain unpaired electrons. Thus
D)
The
The MOT is as shown below,
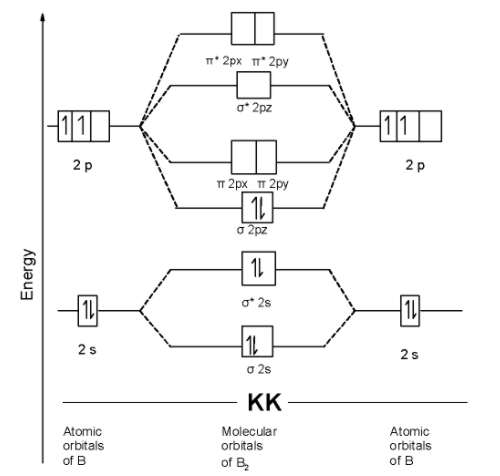
We can write the molecular orbital configuration of
Since the molecular orbitals theory does not contain the unpaired electron. Thus
Thus, from here we know that only
Hence, (C) is the correct option.
Note: Note that, in atoms like
Complete step by step solution:
The MOT diagram is drawn in such a way that we do not consider the s and p orbital mixing. Therefore the order of orbitals is not changed.
Let’s first draw the MOT of all molecules given in the problem.
A)
The
The MOT is as shown below,

First of all, we can write the molecular orbital configuration of
Since, here all molecular orbitals contain the two electrons. These are all paired electrons. Thus
B)
The

We can write the molecular orbital configuration of
Since, here all molecular orbitals contain the two electrons. These are all paired electrons. Thus
C)
The
The MOT is as shown below,

we can write the molecular orbital configuration of
Since the pi molecular orbitals contain unpaired electrons. Thus
D)
The
The MOT is as shown below,

We can write the molecular orbital configuration of
Since the molecular orbitals theory does not contain the unpaired electron. Thus
Thus, from here we know that only
Hence, (C) is the correct option.
Note: Note that, in atoms like
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE




