
An infinite line charge of uniform electric charge density
(A)
(B)
(C)
(D)





Answer
490.5k+ views
2 likes
Hint : In this solution, we will determine the electric field due to a line charge at a distance equal to the radius of the cylinder. The current density generated in the cylinder will be determined using ohm’s law. The current in the
Formula used: In this solution, we will use the following formula:
-Electric field due to a line charge:
- Ohm’s law:
Complete step by step answer
Let us start by calculating the current density in the cylinder. Since the electric field due to a line charge inside the cylinder will be
We can determine the charge density as
Now we know that the current density is the ratio of the current in the circuit
Substituting the value of current density this in the above equation, we get
Since
The charge in the line charge can be calculated as the product of line charge density and the length as
So, we can write
Now the length of the line remains constant so the variable in the above equation must be the line charge density which varies with time.
Integrating the above equation with time, we get
Multiplying both sides by
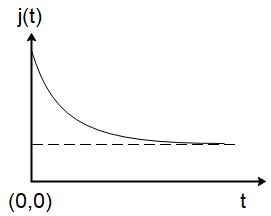
Hence the graph of current density with time is exponentially decaying with time which corresponds to option (A).
Note
In this solution, it is difficult to confer that the line charge density will vary with time which is why we solve the equations to see that the current density could be varying with time only the line charge density is changing. We must also be aware of the general behaviour of different functions when plotted with respect to time.
Formula used: In this solution, we will use the following formula:
-Electric field due to a line charge:
- Ohm’s law:
Complete step by step answer
Let us start by calculating the current density in the cylinder. Since the electric field due to a line charge inside the cylinder will be
We can determine the charge density as
Now we know that the current density is the ratio of the current in the circuit
Substituting the value of current density this in the above equation, we get
Since
The charge in the line charge can be calculated as the product of line charge density and the length as
So, we can write
Now the length of the line remains constant so the variable in the above equation must be the line charge density which varies with time.
Integrating the above equation with time, we get
Multiplying both sides by
Hence the graph of current density with time is exponentially decaying with time which corresponds to option (A).
Note
In this solution, it is difficult to confer that the line charge density will vary with time which is why we solve the equations to see that the current density could be varying with time only the line charge density is changing. We must also be aware of the general behaviour of different functions when plotted with respect to time.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

What are saprophytes Give two examples class 12 biology CBSE

How do you convert from joules to electron volts class 12 physics CBSE

Mirage is a phenomena due to A Refraction of light class 12 physics CBSE

Why did French artist Frederic sorrieu prepare a series class 12 social science CBSE

What is Nutrition Explain Diff Type of Nutrition ?




