
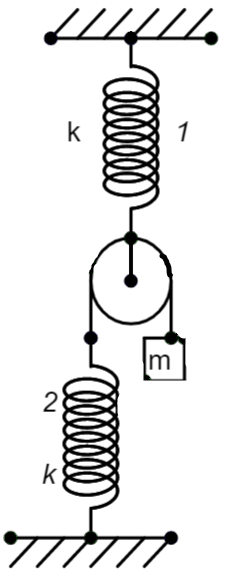
All springs, string, and pulley shown in the figure are light, initially when both the strings were in their natural state, the system was released from the rest. The maximum displacement of the block is

Answer
492.6k+ views
Hint: We need to understand the relation between the two springs on a pulley and the mass hanging on the system with the velocity it may attain once the system is set to motion. The relation can get us the idea of the unknown variable in the problem.
Complete step-by-step solution
We are given a system of springs as given in the figure. We know that the mass ‘m’ hanging from the pulley can extend both the springs. The extension of each of the springs is dependent on the position of the spring in the arrangement.
We know that the extension of spring 1 will be the result of the mass and the extension of spring 2. If ‘a’ is the extension of spring 1 in the system and ‘b’ be the extension on spring 2. When spring 2 is extended, the mass goes in a downward direction for ‘b’ displacement. When spring 2 is released, the total displacement will be twice its displacement, thus becoming ‘2b’.
The mass displaces in both directions with the same quantity as that of spring 1.
The net displacement of the mass can be given as –
Now, let us balance the force due to the mass and the force due to the extension of the spring in the two cases. The springs have a spring constant of ‘k’ and a mass ‘m’ is attached to the pulley connected to spring 2 through a wire.
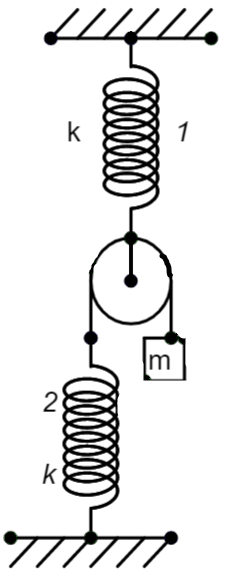
For the spring 1 –
For the spring 2 –
Now, we can find the total displacement from the equation for ‘y’ as –
But we are already given that the total displacement ‘y’ as –
From the above two relations we can conclude that –
The required value for ‘x’ is 1.
This is the required solution.
Note: The springs when attached to masses undergo extension due to the force due to the mass of the body. This extension is directly proportional to the mass hung on the spring as we have seen in this problem. We have to keep this point in mind while solving these.
Complete step-by-step solution
We are given a system of springs as given in the figure. We know that the mass ‘m’ hanging from the pulley can extend both the springs. The extension of each of the springs is dependent on the position of the spring in the arrangement.
We know that the extension of spring 1 will be the result of the mass and the extension of spring 2. If ‘a’ is the extension of spring 1 in the system and ‘b’ be the extension on spring 2. When spring 2 is extended, the mass goes in a downward direction for ‘b’ displacement. When spring 2 is released, the total displacement will be twice its displacement, thus becoming ‘2b’.
The mass displaces in both directions with the same quantity as that of spring 1.
The net displacement of the mass can be given as –
Now, let us balance the force due to the mass and the force due to the extension of the spring in the two cases. The springs have a spring constant of ‘k’ and a mass ‘m’ is attached to the pulley connected to spring 2 through a wire.

For the spring 1 –
For the spring 2 –
Now, we can find the total displacement from the equation for ‘y’ as –
But we are already given that the total displacement ‘y’ as –
From the above two relations we can conclude that –
The required value for ‘x’ is 1.
This is the required solution.
Note: The springs when attached to masses undergo extension due to the force due to the mass of the body. This extension is directly proportional to the mass hung on the spring as we have seen in this problem. We have to keep this point in mind while solving these.
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




