
A wire of length
A.1 W
B.
C.
D.
Answer
487.5k+ views
Hint: Power is the rate at which work is done. It is the amount of energy per unit of time that is consumed. The power unit is the Joule per second (J/s), known as the Watt (in honor of the steam engine's eighteenth-century developer, James Watt). Calculate force acting on the wire and then take out the power.
Formula used:
Complete Step-by-Step solution:
Power is the work performed in a time unit. In other words, power is a measure of how quickly work can be done. Work is the energy needed to apply a force to move a specific distance from an object, where strength is parallel to the displacement. Power is the rate at which that job is performed.
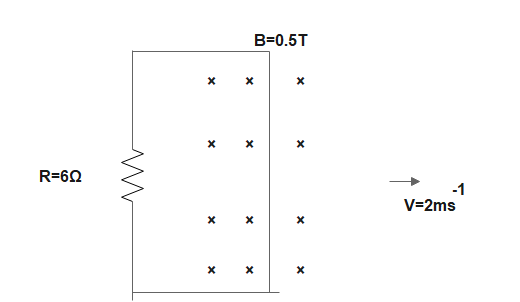
Rate of work
But
Also,
The rate at which work is being done to keep the wire moving at constant speed is
Hence, the correct option is (C).
Note:
The work is a product of the external force and the distance it moves applied to an object or system. Power is a measure of the amount of work per unit of time that is done. In a Force-Distance curve, the work can be computed as the area under a curve. The net work done on an object is equal to the shift in kinetic energy of the object. The alternating-current power in watts delivered to a load by an amplifier is also called the work-energy principle.
Formula used:
Complete Step-by-Step solution:
Power is the work performed in a time unit. In other words, power is a measure of how quickly work can be done. Work is the energy needed to apply a force to move a specific distance from an object, where strength is parallel to the displacement. Power is the rate at which that job is performed.

Rate of work
But
Also,
The rate at which work is being done to keep the wire moving at constant speed is
Hence, the correct option is (C).
Note:
The work is a product of the external force and the distance it moves applied to an object or system. Power is a measure of the amount of work per unit of time that is done. In a Force-Distance curve, the work can be computed as the area under a curve. The net work done on an object is equal to the shift in kinetic energy of the object. The alternating-current power in watts delivered to a load by an amplifier is also called the work-energy principle.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




