
A uniform rod of mass ‘m’ and length ‘L’ is held horizontally by two vertical strings attached to the two ends. One of the strings is cut. Find the angular acceleration soon after it is cut:
A.
B.
C.
D.
Answer
501.6k+ views
Hint: When the string is cut, the rod will rotate around the other end which is tied to the string. The torque responsible for the rotation is due to the weight of the rod at the centre of the rod. Use the relation between torque and moment of inertia to solve this question.
Formula used:
The torque is given as,
Here, F is the force that causes rotation, r is the position of point of force from the centre of rotation and
The relation between torque and moment of inertia is,
Here, I is the moment of inertia of the rod and
Complete step by step answer:
After one of two strings is cut, the rod will move in a circular path around its other end tied to the string as shown in the figure below.
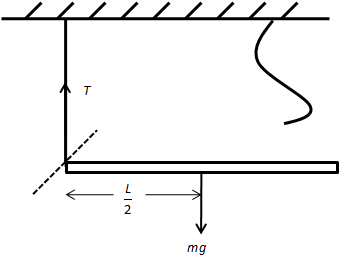
The force which is responsible to rotate the rod around this point is the weight of the rod.
Therefore, the net torque on the rod is due to the weight of the rod which rotates it. The net torque on the rod is,
Here, F is the force that causes rotation, r is the position of point of force from the centre of rotation and
The angle between length of the rod and weight of the rod is
Here, m is the mass of the rod, g is the acceleration due to gravity and L is the length of the rod.
We know the torque acting on the rod of moment of inertia I is,
Here, I is the moment of inertia of the rod and
The moment of inertia of the rod with its rotation axis passing through one of the end is,
Therefore, equation (2) becomes,
From equation (1) and (3), we get,
So, the correct answer is “Option C”.
Note:
The tension in the string also acts on string as one of the forces, but the torque is zero when the force acts at the centre. The angular acceleration and linear acceleration of the rod is different. The linear acceleration is the tangential acceleration of the rod given by
Formula used:
The torque is given as,
Here, F is the force that causes rotation, r is the position of point of force from the centre of rotation and
The relation between torque and moment of inertia is,
Here, I is the moment of inertia of the rod and
Complete step by step answer:
After one of two strings is cut, the rod will move in a circular path around its other end tied to the string as shown in the figure below.

The force which is responsible to rotate the rod around this point is the weight of the rod.
Therefore, the net torque on the rod is due to the weight of the rod which rotates it. The net torque on the rod is,
Here, F is the force that causes rotation, r is the position of point of force from the centre of rotation and
The angle between length of the rod and weight of the rod is
Here, m is the mass of the rod, g is the acceleration due to gravity and L is the length of the rod.
We know the torque acting on the rod of moment of inertia I is,
Here, I is the moment of inertia of the rod and
The moment of inertia of the rod with its rotation axis passing through one of the end is,
Therefore, equation (2) becomes,
From equation (1) and (3), we get,
So, the correct answer is “Option C”.
Note:
The tension in the string also acts on string as one of the forces, but the torque is zero when the force acts at the centre. The angular acceleration and linear acceleration of the rod is different. The linear acceleration is the tangential acceleration of the rod given by
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE




