
A uniform disc of mass m, radius r and a point mass m are arranged as shown in the figure. The acceleration of point mass is : (Assume there is no slipping between the pulley and thread and the disc can rotate smoothly about a fixed horizontal axis passing through its centre and perpendicular to its plane).
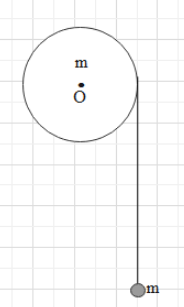
A.
B.
C.
D. None of these

Answer
492k+ views
Hint: To solve the given question we must know about rotational mechanics. Calculate the torque and find the angular acceleration of the disc. With this find the tension in the rope and acceleration of the point mass.
Formula used:
Complete step by step answer:
To the gravitational force acting on the point mass
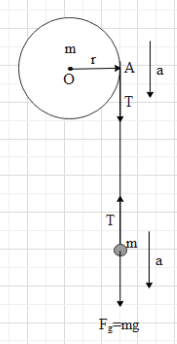
Then the tension in the rope will apply a torque on the disc. As a result, the disc will rotate about the fixed axis at the centre with some angular acceleration.
The magnitude of torque about an axis is given as
where F is the force acting on the disc,
In this case,
The torque is also given as
where I is the moment of inertia of the body about the axis of rotation and
The moment of inertia of a disc of mass m and radius r, about a fixed horizontal axis passing through its centre and perpendicular to its plane is equal to
Substitute the value of I in (ii).
Now equate (i) and (iii).
The linear acceleration of the disc at point A will be
Substitute the value of
Since it is given that there is no slipping between the thread and the disc, the acceleration of the point mass will be
Now, let us find the net force on the point mass m.The gravitational force on the mass is
Therefore, the net force on the point mass is
And from Newton’s second law of motion
Substitute the value of T from (v).
Therefore, the acceleration of the point mass is equal to
Hence, the correct option is C.
Note:A common mistake that can be done while solving the given question is that the students may assume that the tension in the string to be equal to the gravitational force acting on the point mass, i.e.
Formula used:
Complete step by step answer:
To the gravitational force acting on the point mass

Then the tension in the rope will apply a torque on the disc. As a result, the disc will rotate about the fixed axis at the centre with some angular acceleration.
The magnitude of torque about an axis is given as
where F is the force acting on the disc,
In this case,
The torque is also given as
where I is the moment of inertia of the body about the axis of rotation and
The moment of inertia of a disc of mass m and radius r, about a fixed horizontal axis passing through its centre and perpendicular to its plane is equal to
Substitute the value of I in (ii).
Now equate (i) and (iii).
The linear acceleration of the disc at point A will be
Substitute the value of
Since it is given that there is no slipping between the thread and the disc, the acceleration of the point mass will be
Now, let us find the net force on the point mass m.The gravitational force on the mass is
Therefore, the net force on the point mass is
And from Newton’s second law of motion
Substitute the value of T from (v).
Therefore, the acceleration of the point mass is equal to
Hence, the correct option is C.
Note:A common mistake that can be done while solving the given question is that the students may assume that the tension in the string to be equal to the gravitational force acting on the point mass, i.e.
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




