Question

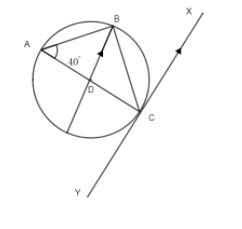
A triangle ABC is inscribed in a circle, and tangent at C to the circle is parallel to the bisector of angle ABC. Find the magnitude of \[\angle CBD\], where D is the point of the intersection of the bisector of angle ABC with AC.

Answer 1

Hint: To solve this question given above, first we will join A and C with the center of the circle. Then we will assume \[\angle BCX\] as x. The triangle OBC obtained will be an isosceles triangle. From this triangle, we will derive a relation between \[\angle BOC\] and \[\angle OCB\]. Now as OC is normal, we will write \[\angle OCX\] as \[{{90}^{\circ }}\]. Then we will derive a relation between \[\angle OCB\] and x with the help of this. With the help of these two relations, we will write \[\angle BOC\] in terms of x. Now \[\angle BOC\] will be double of \[\angle CAB\] because the angle subtended by the chord at center is double the angle subtended at circumference. From here we will get the value of x. Then by using the concept of parallel lines, we will find a relation between x and \[\angle CBD\].

Complete step-by-step answer:
In this question, we are going to assume that the segment ABC is a major segment. We know that if ABC is a major segment then the angle ABC will be less than \[{{90}^{\circ }}\]. If our assumption is wrong then the value of angle ABC will be greater than \[{{90}^{\circ }}\] in the end. So, now we are going to do some construction in the circle. First, we will join the points A and B to the center (O) of the circle. We will then consider angle BCX = x. Thus, after construction the circle will look like:

Now, we know that OB and OC are the radii of the circle. So, they will be equal. So, we can say that triangle OBC is an isosceles triangle. Thus, \[\angle OCB=\angle OBC\]. Now we will write the angle BOC in terms of x. We know that, in a triangle, the sum of all the interior angles is \[{{180}^{\circ }}\]. Thus, in triangle BOC,
\[\Rightarrow \angle COB+\angle OCB+\angle OBC={{180}^{\circ }}\]
\[\Rightarrow \angle COB+2\angle OCB={{180}^{\circ }}\] (\[\angle OCB=\angle OBC\])
\[\angle COB={{180}^{\circ }}-2\angle OCB\] - (i)
Since XY is a tangent at C and OC passes through the center, so OC is the normal of the circle. Also angle between tangent and normal is \[{{90}^{\circ }}\]. So, we will get,
\[\Rightarrow \angle OCX={{90}^{\circ }}\]
\[\Rightarrow \angle OCB+\angle BCX={{90}^{\circ }}\]
\[\Rightarrow \angle OCB={{90}^{\circ }}-\angle BCX\] - (ii)
Now, we will put the value of \[\angle OCB\] from (ii) to (i). After doing this, we will get:
\[\begin{align}
  & \Rightarrow \angle COB={{180}^{\circ }}-2\left( {{90}^{\circ }}-\angle BCX \right) \\
 & \Rightarrow \angle COB={{180}^{\circ }}-{{180}^{\circ }}+2\angle BCX \\
 & \Rightarrow \angle COB=2\angle BCX \\
\end{align}\]
\[\Rightarrow \angle COB=2{{x}^{\circ }}\] - (iii) (\[\angle BCX={{x}^{\circ }}\])
We can also see that, from the properties of an inscribed triangle, \[\angle COB=2\angle CAB\] - (iv).
From (iii) and (iv), we get:
\[\begin{align}
  & \Rightarrow 2\angle CAB=2{{x}^{\circ }} \\
 & \Rightarrow \angle CAB={{x}^{\circ }} \\
 & \Rightarrow {{x}^{\circ }}={{40}^{\circ }} \\
\end{align}\]
Now, we are given that the lines BD and XY are parallel. So, \[\angle DBC=\angle BCX\] (according to alternate interior angles)
\[\begin{align}
  & \Rightarrow \angle DBC={{x}^{\circ }} \\
 & \Rightarrow \angle DBC={{40}^{\circ }} \\
 & \Rightarrow \angle CBD={{40}^{\circ }} \\
\end{align}\]
Now the \[\angle ABC={{80}^{\circ }}\]. It is less than \[{{90}^{\circ }}\]. So our assumption was right. ABC is a major segment. Thus,
\[\Rightarrow \angle CBD={{40}^{\circ }}\]

Note: This question can be solved directly by the alternate segment theorem which says that the angle between the chord and the tangent through one of the end points of the chord is equal to the angle in the alternate segment.