
A square is inscribed in a circle of radius
Answer
492.3k+ views
1 likes
Hint: We will look at a rough diagram to understand what is being asked in the question. We will find the length of the side of the first square in terms of
Complete step-by-step solution
Let us look at a rough diagram, which has the given circle and the first inscribed square in it.
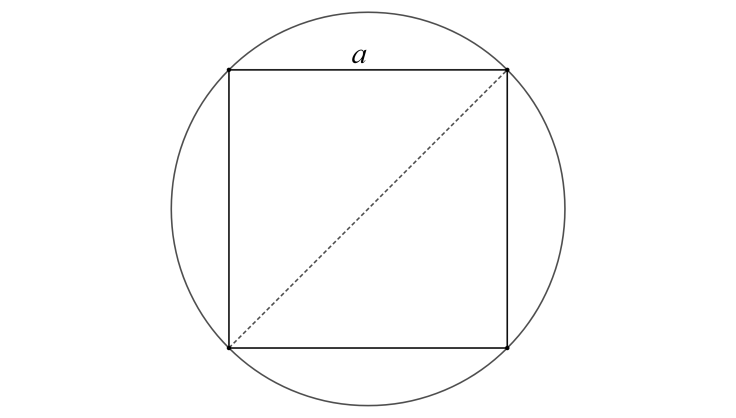
Let the side of the square be
Therefore, the side of the square in terms of the radius of the circle is
Now, we have to inscribe a circle inside the square with side length
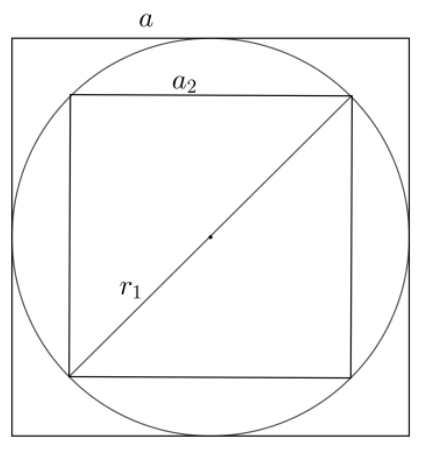
Now, we will inscribe a square of side length
The value of
Now, again we will inscribe a circle inside the square of side length
Next, we will inscribe a square of side length
We have to continue this for '
So, the limit of the sum of areas of the inscribed squares as
Note: In this type of question, it is important that we are able to discern the pattern of the sequence of values we obtain at every step. It is useful to know the concept of geometric progression and its formulae. The key aspect in this is the relation between the diameter of the circle and the diagonal of its inscribed square.
Complete step-by-step solution
Let us look at a rough diagram, which has the given circle and the first inscribed square in it.

Let the side of the square be
Therefore, the side of the square in terms of the radius of the circle is
Now, we have to inscribe a circle inside the square with side length

Now, we will inscribe a square of side length
The value of
Now, again we will inscribe a circle inside the square of side length
Next, we will inscribe a square of side length
We have to continue this for '
So, the limit of the sum of areas of the inscribed squares as
Note: In this type of question, it is important that we are able to discern the pattern of the sequence of values we obtain at every step. It is useful to know the concept of geometric progression and its formulae. The key aspect in this is the relation between the diameter of the circle and the diagonal of its inscribed square.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How do I get the molar mass of urea class 11 chemistry CBSE

How do I convert ms to kmh Give an example class 11 physics CBSE

Where can free central placentation be seen class 11 biology CBSE

What is the molecular weight of NaOH class 11 chemistry CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE




