
A spherical conducting shell of radius R has a charge q. A point charge Q is placed
at its centre. The electrostatic potential at a point P a distance
the centre of the shell is:
A.
B.
C.
D.
Answer
473.7k+ views
1 likes
Hint:The potential at point P at a distance r from the point charge inversely proportional to the distance between the point P and charge q. The potential inside the charged conducting shell remains the same at every point. The potential at point P will be the contribution of potential due to charge placed at the centre of the sphere and the charge distribution on the outer conducting shell.
Formula used:
The potential due to point charge at a distance r is,
Here,
Complete step by step answer:
Let’s draw the situation given in the question as shown in the figure below.
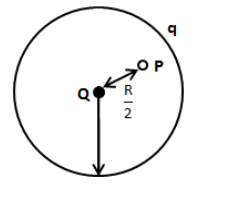
We have the expression for the potential due to point charge at a distance r from the charge
is,
We have given that the position of point P is
shell. Therefore, substituting
We have the expression for the potential inside the charged conducting shell of radius R is,
Here, q is the charge on the sphere.
This potential remains the same at every point inside the sphere. Therefore, the potential at
point P inside the sphere due to charge on the sphere is,
Thus, the potential at point P will the sum of the potential due to point charge Q and
potential inside the charged sphere. Therefore, we can write,
So, the correct answer is option (D).
Note: The potential due to uniform charge distribution of the shell at the point outside the shell is zero. The potential inside the hollow charged conducting shell remains the same at every point due to uniform charge distribution over the surface of the shell. The electric field inside such a shell becomes zero.
Formula used:
The potential due to point charge at a distance r is,
Here,
Complete step by step answer:
Let’s draw the situation given in the question as shown in the figure below.

We have the expression for the potential due to point charge at a distance r from the charge
is,
We have given that the position of point P is
shell. Therefore, substituting
We have the expression for the potential inside the charged conducting shell of radius R is,
Here, q is the charge on the sphere.
This potential remains the same at every point inside the sphere. Therefore, the potential at
point P inside the sphere due to charge on the sphere is,
Thus, the potential at point P will the sum of the potential due to point charge Q and
potential inside the charged sphere. Therefore, we can write,
So, the correct answer is option (D).
Note: The potential due to uniform charge distribution of the shell at the point outside the shell is zero. The potential inside the hollow charged conducting shell remains the same at every point due to uniform charge distribution over the surface of the shell. The electric field inside such a shell becomes zero.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




