
A source of a sound is emitting sound waves in all directions in an absorption less medium. This source is at a distance of 4 m and 16m from points x and y respectively. The ratio of amplitude of waves at points x and y will be
A) 2:4
B) 4:1
C) 4:2
D) 1:4
Answer
495.3k+ views
Hint: We know that sound travels in a form of have from one medium to another. Here we have given a source which is emitting sound in all directions and its distance with respect to any random point x and y is 4 m and 16 m respectively. We have to find the ratio of amplitude at these points x and y.
Formula used:
Complete answer:
Sound waves are the waves which propagate sound energy from one medium to another. Like other waves sound has amplitude which decreases with the distance it travels, therefore when we move away from a source emitting sound waves gradually its intensity decreases and we hear feeble sound or at certain distance we can’t hear the sound which is emitted by the source. Sound waves are also absorbed while travelling from one medium to another or in some cases it is blocked.
According to the question, the source s is emitting sound waves in all directions and the medium in which it is propagating is absorption less and there are two points x and y at some distance from source with respect to which we have to find the amplitude of the sound wave.
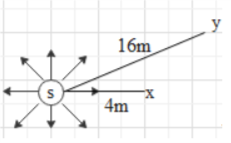
Now, the amplitude of sound wave at any reference point which is placed at distance r from the source is given as
Where A is the amplitude of a sound wave which is emitted from the source.
So the amplitude of sound wave at point x which is 4 m away from the source will be given as
And the amplitude of sound wave at point y which is 16 m away is given as
Hence the ratio of amplitude of waves at points x and y using equation (i) and (ii) will be given as
As we can see the ratio is 4:1, therefore option B is the correct answer.
Note:
In case the medium was not absorption less then while travelling the sound waves would have been absorbed in the way and then we would not be able to apply the formula we used. As due to absorption of the wave the energy will be decreased and so the amplitude of the wave will be decreased.
Formula used:
Complete answer:
Sound waves are the waves which propagate sound energy from one medium to another. Like other waves sound has amplitude which decreases with the distance it travels, therefore when we move away from a source emitting sound waves gradually its intensity decreases and we hear feeble sound or at certain distance we can’t hear the sound which is emitted by the source. Sound waves are also absorbed while travelling from one medium to another or in some cases it is blocked.
According to the question, the source s is emitting sound waves in all directions and the medium in which it is propagating is absorption less and there are two points x and y at some distance from source with respect to which we have to find the amplitude of the sound wave.

Now, the amplitude of sound wave at any reference point which is placed at distance r from the source is given as
Where A is the amplitude of a sound wave which is emitted from the source.
So the amplitude of sound wave at point x which is 4 m away from the source will be given as
And the amplitude of sound wave at point y which is 16 m away is given as
Hence the ratio of amplitude of waves at points x and y using equation (i) and (ii) will be given as
As we can see the ratio is 4:1, therefore option B is the correct answer.
Note:
In case the medium was not absorption less then while travelling the sound waves would have been absorbed in the way and then we would not be able to apply the formula we used. As due to absorption of the wave the energy will be decreased and so the amplitude of the wave will be decreased.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹34,851 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




