
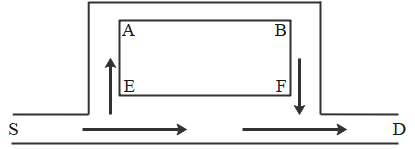
A sound wave starting from source S, follows two paths SEFD and SEABFD. If AB =
A.
B.
C.
D.

Answer
501k+ views
Hint: This question is related to interference of sound waves. The wave generated from source S travels different paths and interferes at D. We will use the condition of constructive interference to obtain value of length
Formula used:
For maxima
Complete step by step answer:
When waves from two coherent sources superimpose, interference occurs. Where both waves interfere constructively, maximum is observed and where the waves interfere destructively minimum is obtained. Interference will be constructive or destructive depending on the path difference between the waves.
If path difference between the waves is an integral multiple of wavelength then both waves interfere constructively and maximum is obtained and if path difference is an odd multiple of half of the wavelength, both waves interfere destructively and minima is obtained. Mathematically,
For maxima
For minima
As given, both the waves originated from the same source but travel different path lengths and interfere at D. Wave travelling along path SEABFD will travel more distance as compared to wave travelling along SEFD. Since at D maximum sound is heard, it means both waves have interfered constructively.
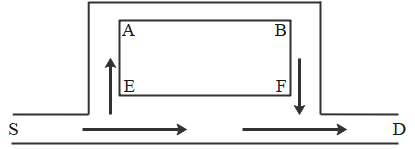
Path length difference between wave travelling along path SEABFD and wave travelling along SEFD is
Since,
It is given that, AE = BF =
Applying the condition of maxima, we get
Wavelength of wave is
This implies that
Therefore, option D is correct.
Note:
When waves from two coherent sources superimpose, interference occurs. Where both waves interfere constructively, maximum is observed and where the waves interfere destructively minimum is obtained. Interference will be constructive or destructive depending on the path difference between the waves.
Formula used:
For maxima
Complete step by step answer:
When waves from two coherent sources superimpose, interference occurs. Where both waves interfere constructively, maximum is observed and where the waves interfere destructively minimum is obtained. Interference will be constructive or destructive depending on the path difference between the waves.
If path difference between the waves is an integral multiple of wavelength then both waves interfere constructively and maximum is obtained and if path difference is an odd multiple of half of the wavelength, both waves interfere destructively and minima is obtained. Mathematically,
For maxima
For minima
As given, both the waves originated from the same source but travel different path lengths and interfere at D. Wave travelling along path SEABFD will travel more distance as compared to wave travelling along SEFD. Since at D maximum sound is heard, it means both waves have interfered constructively.

Path length difference between wave travelling along path SEABFD and wave travelling along SEFD is
Since,
It is given that, AE = BF =
Applying the condition of maxima, we get
Wavelength of wave is
This implies that
Therefore, option D is correct.
Note:
When waves from two coherent sources superimpose, interference occurs. Where both waves interfere constructively, maximum is observed and where the waves interfere destructively minimum is obtained. Interference will be constructive or destructive depending on the path difference between the waves.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




