Question

A small particle of mass m is projected at an angle\[\theta \] with the x-axis with an initial velocity \[{{v}_{0}}\] in the XY plane as shown in the figure. At a time \[t<\dfrac{{{v}_{0}}\sin \theta }{g}\], what is the angular momentum of the particle?
                       

Answer 1

Hint: We need to understand the different physical parameters involved in a projectile motion to correctly interpret the relation between each of them. We can use the given information on velocity to find the angular momentum of the projectile particle.

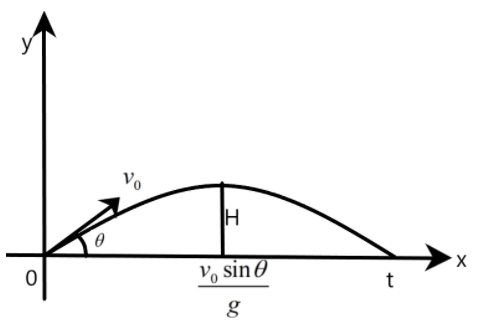
Complete answer: We know that for any projectile motion on earth, the acceleration that causes the motion to be possible is the vertically downward acting acceleration due to the gravity. At the topmost point, when the projectile is at its maximum height, we can derive the time of flight up to that point as –
\[\begin{align}
  & v=u+at \\
 & 0={{v}_{0}}\sin \theta -gt \\
 & \Rightarrow t=\dfrac{{{v}_{0}}\sin \theta }{g} \\
\end{align}\]
We are asked to find the angular momentum of the projectile at any time lesser than this time, which is at the highest point of the projectile.
We can find the velocity of the projectile before this point from the equations of motion as –
\[\begin{align}
  & \vec { u } ={{v}_{0}}\cos \theta \widehat{i}+{{v}_{0}}\sin \theta \widehat{j} \\
 & v=u+at \\
 & \Rightarrow \vec { v } ={{v}_{0}}cos\theta \widehat{i}+{{v}_{0}}\sin \theta \widehat{j}-gt\widehat{j} \\
 & \Rightarrow \vec { v } ={{v}_{0}}\cos \theta \widehat{i}+({{v}_{0}}\sin \theta -gt)\widehat{j} \\
\end{align}\]

We know that from the equations of motion –
\[S=ut+\dfrac{1}{2}a{{t}^{2}}\]
Along the y-axis, we can write it as –
\[\begin{align}
  & S=ut+\dfrac{1}{2}a{{t}^{2}} \\
 & \Rightarrow y={{v}_{0}}\sin \theta t-\dfrac{1}{2}g{{t}^{2}} \\
\end{align}\]
Now, we know that the angular momentum is defined as the cross product of the linear momentum and the radial distance between the points of action. We can find the radial distance as the distance along the y-axis and the distance along the x-axis. It is given by –
\[\ \vec { r } ={{v}_{0}}\cos \theta \widehat{ti}+({{v}_{0}}\sin \theta t-\dfrac{1}{2}g{{t}^{2}})\widehat{j}\]
Now, we can find the angular momentum using the formula as –
\[\begin{align}
& \vec { L } =\vec { r } \times \vec { p } \\
& \Rightarrow \vec { L } =[{{v}_{0}}\cos \theta t\widehat{i}+({{v}_{0}}\sin \theta t-\dfrac{1}{2}g{{t}^{2}})\widehat{j}]\times m[{{v}_{0}}\cos \theta \widehat{i}+({{v}_{0}}\sin \theta -gt)\widehat{j}] \\
& \therefore \vec { L } =-\dfrac{1}{2}m{{v}_{0}}g\cos \theta {{t}^{2}}\overset\frown{k} \\
\end{align}\]
So, this is the required angular momentum of the particle.

Note:
The angular momentum of a particle changes its direction keeping the magnitude constant when the particle starts to move along the downward direction after half the time of flight. That is because we are asked to find the angular momentum of the first half alone.