
A small ball of mass ′
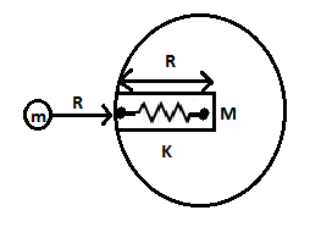

Answer
499.8k+ views
2 likes
Hint: Consider the law of conservation of energy to solve this question. Initially, the ball has gravitational potential energy. When the ball is released, as while falling down, it attains kinetic energy. Once it touches the spring, the spring compresses, due to which the ball attains elastic potential energy. When the ball is inside the groove, gravitational potential energy also acts on the ball. Hence, according to the law of conservation of energy, the initial energy on the ball and final energy on the ball are equal. Here, the initial gravitational potential energy is equal to the sum of final gravitational potential energy and the elastic potential energy. Using this we can find out the value of the spring constant.
Formula used:
Complete step-by-step solution:
Given,
Applying conservation energy,
Initially, the ball has a gravitational potential energy and at the final position it has elastic potential energy and final gravitational potential energy. Then,
Initial gravitational potential energy of the ball is given by,
Where,
At any point inside a sphere, the gravitational potential energy is given by;
Where,
Then, final gravitational potential energy of the ball is given by,
Where,
Now, let’s find the elastic potential energy due to spring.
We have,
Where,
Then,
Here,
Then,
Substitute 2, 3 and 4 in equation 1, we get,
The answer is option D.
Note: The gravitational constant is the proportionality constant which is used in Newton’s Law of gravitation, and is denoted by G, with a value
Formula used:
Complete step-by-step solution:

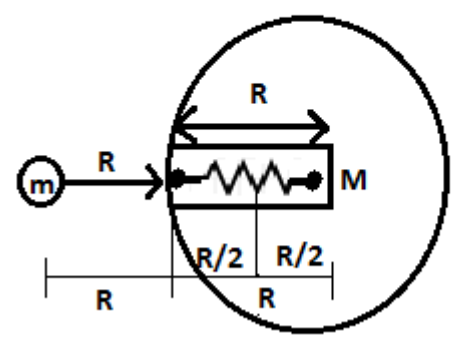
Given,
Applying conservation energy,
Initially, the ball has a gravitational potential energy and at the final position it has elastic potential energy and final gravitational potential energy. Then,
Initial gravitational potential energy of the ball is given by,
Where,
At any point inside a sphere, the gravitational potential energy is given by;
Where,
Then, final gravitational potential energy of the ball is given by,
Where,
Now, let’s find the elastic potential energy due to spring.
We have,
Where,
Then,
Here,
Then,
Substitute 2, 3 and 4 in equation 1, we get,
The answer is option D.
Note: The gravitational constant is the proportionality constant which is used in Newton’s Law of gravitation, and is denoted by G, with a value
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




