
A moving body of mass
Answer
514.2k+ views
1 likes
Hint: For a head on elastic collision, the kinetic energy of the system remains conserved. It means that the total kinetic energy of the system before collision is equal to the total kinetic energy of the system after collision. For calculating the fraction of kinetic energy lost by the colliding particle after collision, we will find the individual kinetic energy of the colliding body before and after the collision.
Formula used:
Conservation of total kinetic energy of the system during an elastic collision,
Complete step by step answer:
Collision, also known as impact, is the sudden and forceful coming together in direct contact of two bodies. A head-on, or direct, collision means that the point of impact is on the straight line connecting the centre of gravity of each of the body.
Two types of collisions can be Elastic collision and Non-elastic collision.
In elastic collision, the total kinetic energy of the two colliding bodies, that is our system, remains conserved. There is no net loss in kinetic energy of the system as a result of an elastic collision. The total system’s kinetic energy before the collision equals the total system’s kinetic energy after the collision.
We are given that a moving body of mass
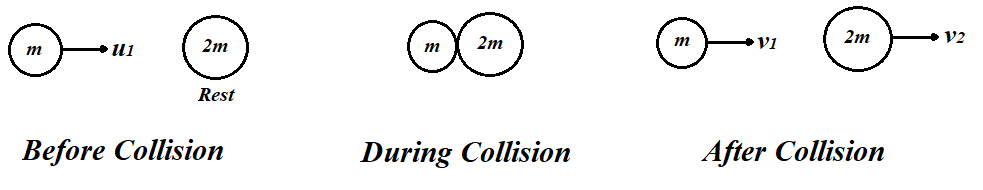
By applying conservation of total kinetic energy of the system during collision, we have,
Where,
Or,
We have,
Therefore,
Now,
Initial kinetic energy of the colliding body is given by,
As,
Therefore,
Final kinetic energy of the colliding body is given by,
As,
Therefore,
Change in Kinetic energy of the colliding body is given by,
We have,
Therefore,
Fractional change in Kinetic energy of the colliding body is given by,
The fraction of kinetic energy lost by the colliding particle after collision is
Note:
In a head on elastic collision, there is no net loss in the total kinetic energy of the system. But, the individual kinetic energies of the two bodies may vary before and after the collision. The fractional change in kinetic energy of a colliding body is expressed as the ratio of change in kinetic energy of the body before and after the collision to the initial kinetic energy of the body before the collision.
Formula used:
Conservation of total kinetic energy of the system during an elastic collision,
Complete step by step answer:
Collision, also known as impact, is the sudden and forceful coming together in direct contact of two bodies. A head-on, or direct, collision means that the point of impact is on the straight line connecting the centre of gravity of each of the body.
Two types of collisions can be Elastic collision and Non-elastic collision.
In elastic collision, the total kinetic energy of the two colliding bodies, that is our system, remains conserved. There is no net loss in kinetic energy of the system as a result of an elastic collision. The total system’s kinetic energy before the collision equals the total system’s kinetic energy after the collision.
We are given that a moving body of mass

By applying conservation of total kinetic energy of the system during collision, we have,
Where,
Or,
We have,
Therefore,
Now,
Initial kinetic energy of the colliding body is given by,
As,
Therefore,
Final kinetic energy of the colliding body is given by,
As,
Therefore,
Change in Kinetic energy of the colliding body is given by,
We have,
Therefore,
Fractional change in Kinetic energy of the colliding body is given by,
The fraction of kinetic energy lost by the colliding particle after collision is
Note:
In a head on elastic collision, there is no net loss in the total kinetic energy of the system. But, the individual kinetic energies of the two bodies may vary before and after the collision. The fractional change in kinetic energy of a colliding body is expressed as the ratio of change in kinetic energy of the body before and after the collision to the initial kinetic energy of the body before the collision.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

A car travels 100 km at a speed of 60 kmh and returns class 11 physics CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE

In tea plantations and hedge making gardeners trim class 11 biology CBSE

A difference between diffusion and osmosis is a A semipermeable class 11 chemistry CBSE




