
A motor boat going downstream in a flowing river overcame a raft at a point P. One hour later it turned back and after some time passed the raft at a distance
Answer
497.1k+ views
Hint: Since the boat is travelling in the direction of river flow, the velocity of boat will be affected by the river velocity. While returning, velocity of boat will be the difference in boat velocity and water velocity. By making equations using the equation for velocity, substituting the values accordingly and equating them we can find the time taken by boat to meet the raft after reverting.
Formula used:
Complete answer:
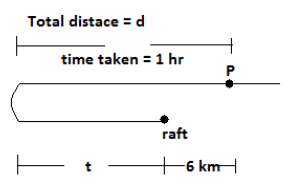
Let
We have,
Velocity,
Then,
Assume that, boat meets the raft in time
During the total time
Substitute equation 3 and 1 in equation 2. We get,
Then,
Time taken by the boat to meet the raft is
Therefore, the correct option is option (B).
Note:
If a boat is travelling along the direction of a river flow, the net velocity of the boat will be the sum of its individual velocity and river velocity. And if it is travelling against the river flow, the net velocity of the boat will be different in the boat velocity and river velocity.
Formula used:
Complete answer:

Let
We have,
Velocity,
Then,
Assume that, boat meets the raft in time
During the total time
Substitute equation 3 and 1 in equation 2. We get,
Then,
Time taken by the boat to meet the raft is
Therefore, the correct option is option (B).
Note:
If a boat is travelling along the direction of a river flow, the net velocity of the boat will be the sum of its individual velocity and river velocity. And if it is travelling against the river flow, the net velocity of the boat will be different in the boat velocity and river velocity.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE




