Question

A mapping is selected at random from all the mappings defined on the set. A consisting of three distinct elements. The probability that the mapping selected is one to one is
A.$\dfrac{1}{9}$
B.$\dfrac{1}{3}$
C.$\dfrac{1}{4}$
D.$\dfrac{2}{9}$

Answer 1

Hint:
If A and B are two non-empty sets, then a relation ‘f’ from set A to set B is said to be a function or mapping,
If every element of set A is associated with unique element of set B,
The mapping or function ‘f’ from A to B is denoted by f:A→A,
If f is a function from A to B and x ϵ A, then f(x) ϵ B where f(x) is called the image of x under f and x is called the preimage of f(x) under ‘f’.
Thus, for a mapping from A to B,
A and B should be non-empty.
Each element of A should have an image in B.
No element of ‘A’ should have more than one image in ‘B’.
Find the number of one-one maps and the total number of mappings from A to A.
$\therefore {\text{Required Probability = }}\dfrac{{{\text{Number of one - one map}}}}{{{\text{Total number of maps}}}}$

Complete step by step solution:
∴ A has 3-elements. Let it be a, b, c
∴ f :A→A

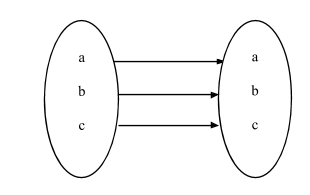
∴Number of one to one mapping $ = 3 \times 2 \times 1$
$ = 6$
And total number of mapping $ = 3 \times 3 \times 3$
$ = 27$
∴ Probability that the mapping is one to one is $ = \dfrac{{{\text{Number of one to one maps}}}}{{{\text{Total number of maps}}}}$
$ = \dfrac{2}{9}$
∴ Option (D) is correct

Note:
Every mapping is a relation but every relation may not be a mapping. If a set has n-elements and a mapping f is defined on A. Therefore, number of one-to-one mapping is \[\left| \!{\underline {\,
  n \,}} \right. \]and the total number of mappings is\[{\left( n \right)^n}\]. Some important points to be keep in mind:
Two or more elements of A may have the same image in B.
f: x →y means that under the function of ‘f’ from A to B, an element x of A has image y in B.
It is necessary that every f image is in B but there may be some elements in B which are not f images of any element of A.