
A freely moving piston divides a vertical cylinder closed at both ends, into two parts each containing
Answer
433.2k+ views
Hint: Since the problem involves gases in a closed cylinder there is a pressure and a corresponding force associated with it. The equation based on the concept of equilibrium is applied in order to determine the forces exerted by the gases on the piston considering the mass of the piston as well which will give the equations relating the pressures at the two conditions of temperatures. The ideal gas equation is then applied in order to determine the required temperature.
Formula used:
The ideal gas equation is given by:
Where,
Complete step by step answer:
When a piston is placed inside a cylinder which is closed at both ends then the piston divides the air or the gas inside the cylinder so that there is some amount of gas present above the piston and the remaining amount of gas present below the piston. Hence, the concept of pressure comes into picture because the molecules of the gas particles present above and below the piston are bound to exert a force on the piston because pressure is said to be the force exerted by a gas per unit area
The diagram below illustrates how the piston has divided the cylinder into two portions:
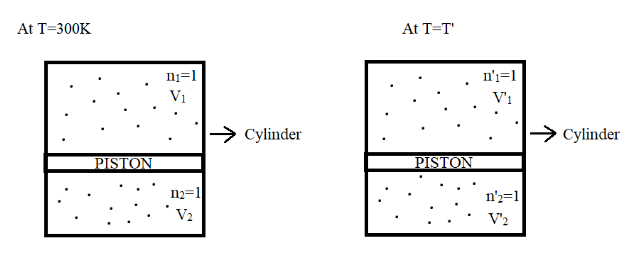
The problem takes two temperature conditions into consideration, that is, one at
There will be a certain amount of force that will be exerted on the piston by the air molecules present in the upper part of the cylinder. Similarly there will be a force that is exerted by the piston on the lower portion of the gas molecules as well. Hence, to balance out these forces an upward force will be exerted by the gas molecules present in the lower portion of the cylinder. This is where the concept of equilibrium comes into picture.
Therefore, by the concept of equilibrium the force exerted by the upper portion and the force applied by the piston will be equivalent to the force exerted by the lower portion of the cylinder.From the above discussed concept we can construct an equation:
Where,
Hence by writing the above equation in terms of pressure and area we get:
Where,
Clearly, the force exerted by the piston is simply the gravitational force. Here,
Similarly let us now take the condition in which the temperature is changed. The equation for the force exerted by the gases at this changed temperature will be different since the gases will have more energy now and hence the pressure of the gases at this condition also changes. The equation becomes:
Since the same piston is being used with a variation of only the temperature conditions the area and the force exerted by the piston will remain unchanged.
Since (
Since the area of the piston is the same everywhere we get:
The common terms are cancelled out to get:
This is the equation relating the pressures of the gases at the two temperature conditions.
Now, let us come to the volume of these gases at the two temperature conditions. For the temperature condition where
Hence we get the relation,
When the temperature changes it changes the volume of the gases as well. For the changed temperature condition, the volume of the upper part, say
We now apply the ideal gas equation. The equation is as follows:
By rearranging the terms we get:
The above ideal gas equation is now substituted in equation (
Where,
Here, the
By cancelling out the common terms we and substituting the value of
We now substitute the equations (
We now determine an equation relating the volumes of the gases in the second temperature condition with the volumes of the gases at
This means that the total volume of the cylinder at
By solving out the equation we get:
By substituting equation (
By taking the common terms out we get:
The common term
The negative signs are cancelled out to get:
We solve out the equation by cross multiplying the terms to get the value of
This is the second temperature condition which we were required to find.
Note: The volume of the gas is said to be the volume of the container in which it is confined in. The common mistake in this problem is that the force exerted by the two portions of the gas particles are equated and the force of the piston is not considered, which is wrong. The piston also has a mass and hence the gravitational force is exerted on the gas molecules.
Formula used:
The ideal gas equation is given by:
Where,
Complete step by step answer:
When a piston is placed inside a cylinder which is closed at both ends then the piston divides the air or the gas inside the cylinder so that there is some amount of gas present above the piston and the remaining amount of gas present below the piston. Hence, the concept of pressure comes into picture because the molecules of the gas particles present above and below the piston are bound to exert a force on the piston because pressure is said to be the force exerted by a gas per unit area
The diagram below illustrates how the piston has divided the cylinder into two portions:

The problem takes two temperature conditions into consideration, that is, one at
There will be a certain amount of force that will be exerted on the piston by the air molecules present in the upper part of the cylinder. Similarly there will be a force that is exerted by the piston on the lower portion of the gas molecules as well. Hence, to balance out these forces an upward force will be exerted by the gas molecules present in the lower portion of the cylinder. This is where the concept of equilibrium comes into picture.
Therefore, by the concept of equilibrium the force exerted by the upper portion and the force applied by the piston will be equivalent to the force exerted by the lower portion of the cylinder.From the above discussed concept we can construct an equation:
Where,
Hence by writing the above equation in terms of pressure and area we get:
Where,
Clearly, the force exerted by the piston is simply the gravitational force. Here,
Similarly let us now take the condition in which the temperature is changed. The equation for the force exerted by the gases at this changed temperature will be different since the gases will have more energy now and hence the pressure of the gases at this condition also changes. The equation becomes:
Since the same piston is being used with a variation of only the temperature conditions the area and the force exerted by the piston will remain unchanged.
Since (
Since the area of the piston is the same everywhere we get:
The common terms are cancelled out to get:
This is the equation relating the pressures of the gases at the two temperature conditions.
Now, let us come to the volume of these gases at the two temperature conditions. For the temperature condition where
Hence we get the relation,
When the temperature changes it changes the volume of the gases as well. For the changed temperature condition, the volume of the upper part, say
We now apply the ideal gas equation. The equation is as follows:
By rearranging the terms we get:
The above ideal gas equation is now substituted in equation (
Where,
Here, the
By cancelling out the common terms we and substituting the value of
We now substitute the equations (
We now determine an equation relating the volumes of the gases in the second temperature condition with the volumes of the gases at
This means that the total volume of the cylinder at
By solving out the equation we get:
By substituting equation (
By taking the common terms out we get:
The common term
The negative signs are cancelled out to get:
We solve out the equation by cross multiplying the terms to get the value of
This is the second temperature condition which we were required to find.
Note: The volume of the gas is said to be the volume of the container in which it is confined in. The common mistake in this problem is that the force exerted by the two portions of the gas particles are equated and the force of the piston is not considered, which is wrong. The piston also has a mass and hence the gravitational force is exerted on the gas molecules.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

1 litre is equivalent to A 1000mL B 100cm3 C 10mL D class 11 physics CBSE

A car travels 100 km at a speed of 60 kmh and returns class 11 physics CBSE

Name the Largest and the Smallest Cell in the Human Body ?

Explain zero factorial class 11 maths CBSE

In tea plantations and hedge making gardeners trim class 11 biology CBSE




