
A cylinder of mass
(A)
(B)
(C)
(D)

Answer
478.2k+ views
Hint Hint: To solve this question, we need to use the theorem of conservation of angular momentum. For that, we have to equate the angular momentum of the cylinder about the edge of the step, before and after the collision.
Formula used: The formulae used to solve this question are
(A)
(B)
Complete step-by-step solution:
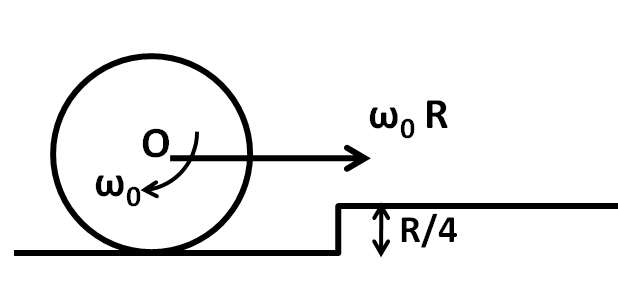
The rolling cylinder will interact with the step at the point P as shown in the below figure.

As we can see that a normal force
Initial angular momentum:
In the above figure, the cylinder is rotating with the angular velocity of
The perpendicular distance of the velocity vector from the point P from the above figure can be given by
Also, we know that the moment of inertia of a cylinder about its natural axis is given by
Putting (2) and (3) in (1) we get
On simplifying, we get
Final angular momentum:
Let the final angular velocity of the cylinder be
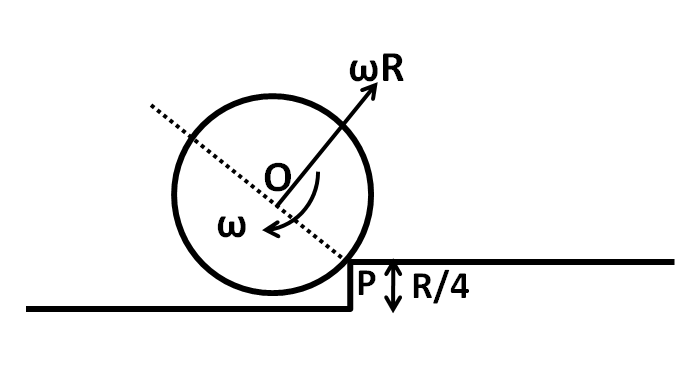
Since it is given that no slipping occurs on the edge, the translational velocity of the centre of mass of the cylinder is given by
Since the cylinder is translating with velocity
In this case, the perpendicular distance of P from the velocity vector is equal to the radius, as can be seen in the above figure. So we can put
Putting (3) and (5) in the above equation, we get
On simplifying we get
Now, by the conservation of angular momentum, we equate (4) and (6) to get
Cancelling
Multiplying with
Hence, the correct answer is option A.
Note: We can also attempt this question using the concept of instantaneous axis of rotation. The given cylinder will have a pure rotation about the instantaneous axis passing through P and perpendicular to the plane of the cylinder. So its angular momentum about P will be given by
Formula used: The formulae used to solve this question are
(A)
(B)
Complete step-by-step solution:
The rolling cylinder will interact with the step at the point P as shown in the below figure.

As we can see that a normal force
Initial angular momentum:
In the above figure, the cylinder is rotating with the angular velocity of
The perpendicular distance of the velocity vector from the point P from the above figure can be given by
Also, we know that the moment of inertia of a cylinder about its natural axis is given by
Putting (2) and (3) in (1) we get
On simplifying, we get
Final angular momentum:
Let the final angular velocity of the cylinder be

Since it is given that no slipping occurs on the edge, the translational velocity of the centre of mass of the cylinder is given by
Since the cylinder is translating with velocity
In this case, the perpendicular distance of P from the velocity vector is equal to the radius, as can be seen in the above figure. So we can put
Putting (3) and (5) in the above equation, we get
On simplifying we get
Now, by the conservation of angular momentum, we equate (4) and (6) to get
Cancelling
Multiplying with
Hence, the correct answer is option A.
Note: We can also attempt this question using the concept of instantaneous axis of rotation. The given cylinder will have a pure rotation about the instantaneous axis passing through P and perpendicular to the plane of the cylinder. So its angular momentum about P will be given by
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE




