Question

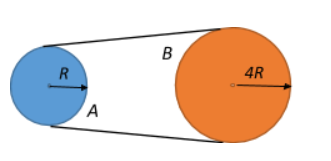
A continuous conveyor belt passes over two discs A and B of radii R and 4R respectively. They start from rest. A has angular acceleration of $\dfrac{\pi}{4}\;rads^{-2}$. What is the angular velocity of the disk B at the end of 32 seconds?

Answer 1

Hint: For the conveyer to work effectively, the (linear) velocity with which the belt moves between the two discs must be the same as the two discs. This means that both the discs have the same linear velocity. To this end, first find the angular velocity and then the linear velocity of disc A using the angular acceleration given to us. Then, find the expression for the angular velocity of disc B. Equate the two to arrive at the angular velocity for disc B after the given time.
Formula Used:
Angular acceleration $\alpha = \dfrac{\omega}{t}$
Angular velocity $\omega = \dfrac{v}{r}$

Complete answer:
We are given with two discs A and B that constitute a conveyor belt system.
Disc A has a radius of $r_A = R$ and an angular acceleration $\alpha_A = \dfrac{\pi}{4}\;rads^{-2}$ and disc B has a radius of $r_B = 4R$. We are required to find the angular velocity of disk B at the end of time $t=32\;s$.
Now, we know that a conveyor system such as the one in the question requires both the disks to work in tandem with each other to perform appropriately. In such a case, the rate at which the conveyor belt moves between the disks must be uniform. This means that the belt moves with the same linear velocity at B, as it does at A.
Let us begin by first calculating the linear velocity of the belt at disc A.
We know that angular acceleration is the rate of change of angular velocity with time., i.e,
$\alpha = \dfrac{\omega}{t}$
From the above expression, we have the angular velocity of A to be:
$\omega_A = \alpha_A \times t = \dfrac{\pi}{4} \times 32 = 8\pi\;rads^{-1}$
Now, linear velocity is nothing but the tangential velocity component of a rotating body at a radial distance. This is quantized as:
$v = r\omega$ and in our case,
$v_A = r_A \times \omega_A = R \times 8\pi = 8\pi R$
Similarly, $v_B = r_B \times \omega_B = 4R \times \omega_B = 4R\omega_B$
We have previously established that $v_A = v_B$, so we equate the above two expressions:
$4R\omega_B = 8\pi R$
$\Rightarrow \omega_B = \dfrac{8\pi R}{4R}$
$\Rightarrow \omega_B = 2\pi\; rads^{-1}$
Therefore, the angular velocity of the disk B at the end of 32 seconds is found to be $2\pi\;rads^{-1}$, which means that it completes one whole rotation in one second.

Note:
It is important to understand the distinction between linear velocity and angular velocity and establish a relationship between the two. Linear velocity is generally associated with linear displacements or linear motion which is any motion that occurs along a straight line in one direction, whereas angular velocity is associated with angular displacements or rotatory motion which is any motion where a body moves in a circular path about an axis of rotation.
Both describe the rate at which the displacement of a body occurs, except the nature of such displacements are specific to the type of motion it executes. Linear motion is measured in $ms^{-1}$ whereas angular velocity is measured in $rads^{-1}$. Note that though linear velocity is associated with straight line motion, it is also a measurable quantity in circular motion in addition to the angular velocity. The linear velocity at a point is directed tangentially away from the radius of the path, whereas the angular velocity is directed radially.