
A car accelerates from rest at a constant rate
Answer
451.9k+ views
8 likes
Hint: Assuming the maximum velocity, the one relation that relates all the given quantity is
Complete step by step answer:
Let us consider the car accelerates from rest at a constant rate
where, v is the final velocity, u is the initial velocity, a is the constant acceleration and t is the time taken. In this case,
Therefore, we get,
Again, let us consider, the car decelerates from
Therefore, we get,
Drawing the car’s journey on a graph, we get
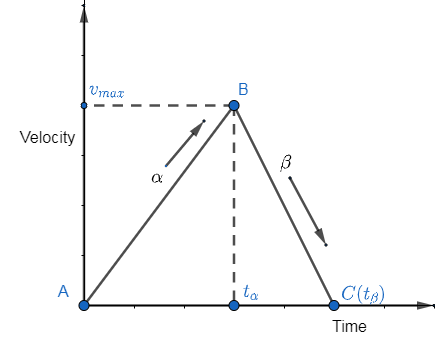
From the above graph, AB is the car's acceleration journey and BC is the decelerated journey.
From the question we know, the entire time taken is t, where
Therefore, substituting this value in the below equation, we get
Now taking out the common term, we get
Taking LCM and solving, we get
Therefore, the correct option is D.
Note: If you look closely into the options, you would notice that they are of different dimensions. Hence, without even doing the sum, by performing a simple dimensional analysis, one can say the correct answer is D. It’s a trick that comes handy in a lot of such sums.
Complete step by step answer:
Let us consider the car accelerates from rest at a constant rate
where, v is the final velocity, u is the initial velocity, a is the constant acceleration and t is the time taken. In this case,
Therefore, we get,
Again, let us consider, the car decelerates from
Therefore, we get,
Drawing the car’s journey on a graph, we get

From the above graph, AB is the car's acceleration journey and BC is the decelerated journey.
From the question we know, the entire time taken is t, where
Therefore, substituting this value in the below equation, we get
Now taking out the common term, we get
Taking LCM and solving, we get
Therefore, the correct option is D.
Note: If you look closely into the options, you would notice that they are of different dimensions. Hence, without even doing the sum, by performing a simple dimensional analysis, one can say the correct answer is D. It’s a trick that comes handy in a lot of such sums.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How do I get the molar mass of urea class 11 chemistry CBSE

How do I convert ms to kmh Give an example class 11 physics CBSE

Where can free central placentation be seen class 11 biology CBSE

What is the molecular weight of NaOH class 11 chemistry CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE




