
A box of mass 1 kg starts to move up on a frictionless inclined plane of inclination 30° with an acceleration
(A)
(B)
(C)
(D)
Answer
505.5k+ views
Hint:
When an object moves on an inclined plane, then the object applies some force on the surface due to the weight of the object. The applied force has two components: the one works along the surface of the plane and the other works perpendicular to the plane.
Complete step-by-step solution:
The mass of the box is:
The angle of the inclined plane is:
The acceleration of the object along the surface of the inclined plane is :
The schematic diagram of the system is given as follows,
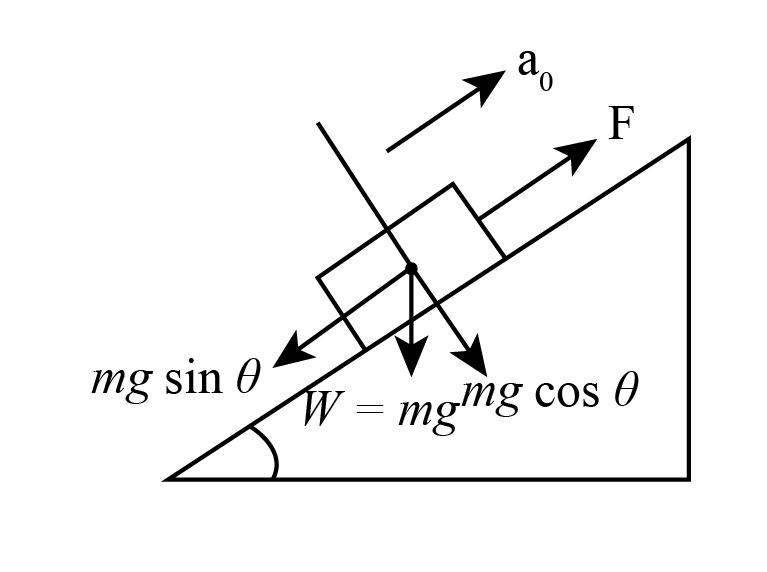
Here we can see that there is a component of the weight, that is working just opposite to the force
- Now we balance the force along the surface of inclined plane,
- Now we put the mass
- Now the component of the force along the surface of the inclined plane due to the weight of the box becomes
As the box slides down with the same acceleration
- Now we will again balance the force along the surface of inclined plane,
- Substitute the value of
Solve further,
Taking The acceleration due to the gravity is:
- Substitute all the values in the above expression,
It is known that
Therefore, the value of
the correct answer is option (C).
Note:
In such a type of question, If the friction is present between the surface of the inclined plane and the object, then we will consider the friction force and this force will act opposite to the direction of the motion of the object.
When an object moves on an inclined plane, then the object applies some force on the surface due to the weight of the object. The applied force has two components: the one works along the surface of the plane and the other works perpendicular to the plane.
Complete step-by-step solution:
The mass of the box is:
The angle of the inclined plane is:
The acceleration of the object along the surface of the inclined plane is :
The schematic diagram of the system is given as follows,

Here we can see that there is a component of the weight, that is working just opposite to the force
- Now we balance the force along the surface of inclined plane,
- Now we put the mass
- Now the component of the force along the surface of the inclined plane due to the weight of the box becomes
As the box slides down with the same acceleration
- Now we will again balance the force along the surface of inclined plane,
- Substitute the value of
Solve further,
Taking The acceleration due to the gravity is:
- Substitute all the values in the above expression,
It is known that
Therefore, the value of
the correct answer is option (C).
Note:
In such a type of question, If the friction is present between the surface of the inclined plane and the object, then we will consider the friction force and this force will act opposite to the direction of the motion of the object.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

A car travels 100 km at a speed of 60 kmh and returns class 11 physics CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE

In tea plantations and hedge making gardeners trim class 11 biology CBSE

A difference between diffusion and osmosis is a A semipermeable class 11 chemistry CBSE




