
A block of mass
(A)
(B)
(C)
(D)

Answer
490.8k+ views
Hint
To solve this question, firstly we have to find the maximum extension of the spring. From there we can get the maximum potential energy of the block, which can be equated to the maximum kinetic energy to get the maximum speed.
Formula Used:
The formula used to solve this question is given by:
Complete step by step answer
The situation is shown in the below diagram
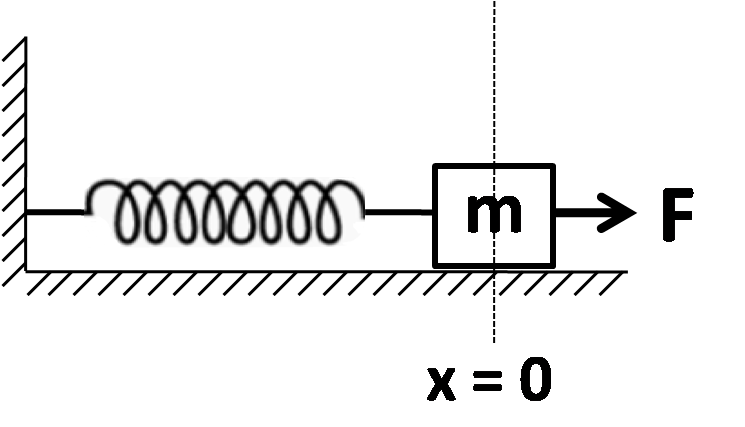
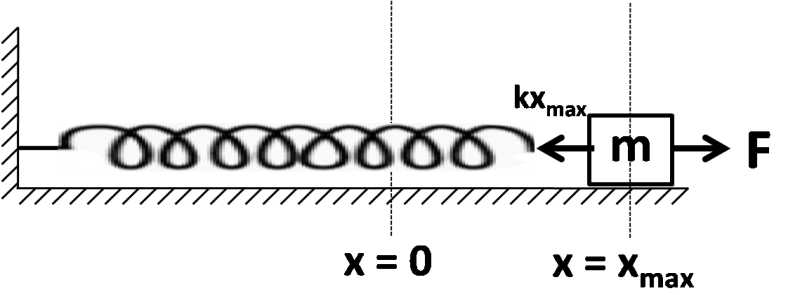
We fix the origin at the initial position of the block. Now, on the application of the force
So, we have from the equilibrium of the block
Or,
Now, we know that the potential energy of a stretched spring is given by
So, the maximum potential energy of the stretched spring is equal to
We also know that the kinetic energy of an object is given by
So, the maximum kinetic energy is
Since there is no friction, the total energy of the block remains constant. Also, we know that when the kinetic energy of the block is maximum, its potential energy is zero and vice-versa.
Therefore we can say that
From (2) and (3) we have
Multiplying both sides by
Substituting the value of
Finally, taking square root we get
Therefore, the maximum speed of the block is equal to
Hence, the correct answer is option (C),
Note
The same question can also be solved using the work-energy theorem. We just need to find the work done by the constant force and the spring force, which is to be equated with the change in kinetic energy, at the extreme position. But the first step to solve these types of questions, by any method is to find out the maximum extension of the spring.
To solve this question, firstly we have to find the maximum extension of the spring. From there we can get the maximum potential energy of the block, which can be equated to the maximum kinetic energy to get the maximum speed.
Formula Used:
The formula used to solve this question is given by:
Complete step by step answer
The situation is shown in the below diagram


We fix the origin at the initial position of the block. Now, on the application of the force
So, we have from the equilibrium of the block
Or,
Now, we know that the potential energy of a stretched spring is given by
So, the maximum potential energy of the stretched spring is equal to
We also know that the kinetic energy of an object is given by
So, the maximum kinetic energy is
Since there is no friction, the total energy of the block remains constant. Also, we know that when the kinetic energy of the block is maximum, its potential energy is zero and vice-versa.
Therefore we can say that
From (2) and (3) we have
Multiplying both sides by
Substituting the value of
Finally, taking square root we get
Therefore, the maximum speed of the block is equal to
Hence, the correct answer is option (C),
Note
The same question can also be solved using the work-energy theorem. We just need to find the work done by the constant force and the spring force, which is to be equated with the change in kinetic energy, at the extreme position. But the first step to solve these types of questions, by any method is to find out the maximum extension of the spring.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




