
A block of mass

Answer
435.6k+ views
Hint: A free body diagram has to be drawn for the block showing the forces act on it. From the diagram, the force is found in terms of the mass of the block. Another force exists here due to the charge on a magnetic field. By equating these forces for the given condition the velocity of the block can be calculated. After getting the velocity the required time can also be found from the equation of motion of the block.
Formula used:
The force
The force occurs due to the magnetic field,
The velocity at time
Complete answer:
The given diagram is,
The diagram has to be modified to show the forces acting on it and also on the system.
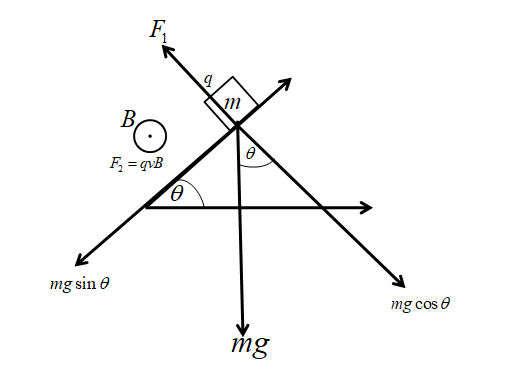
The weight of the block
The force
So,
And due to the magnetic field
These two forces are equal when the block start leaving the incline plane having the effect of the magnetic field,
So,
Now the velocity of the block at time
put the value of
So, the time from start when block loses contact with the surface
Hence, the right answer is in option (D).
Note:
The magnitude of the force on a magnetic field having a charge
Formula used:
The force
The force occurs due to the magnetic field,
The velocity at time
Complete answer:
The given diagram is,

The diagram has to be modified to show the forces acting on it and also on the system.

The weight of the block
The force
So,
And due to the magnetic field
These two forces are equal when the block start leaving the incline plane having the effect of the magnetic field,
So,
Now the velocity of the block at time
put the value of
So, the time from start when block loses contact with the surface
Hence, the right answer is in option (D).
Note:
The magnitude of the force on a magnetic field having a charge
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How do I get the molar mass of urea class 11 chemistry CBSE

How do I convert ms to kmh Give an example class 11 physics CBSE

Where can free central placentation be seen class 11 biology CBSE

What is the molecular weight of NaOH class 11 chemistry CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE




