
A block of mass
(a)
(b)
(c)
(d)
Answer
468k+ views
Hint :We will calculate velocity of block falling on spring from height of
Where,
Where,
Where,
Complete Step By Step Answer:
Given,
Let's imagine a spring is there and a block
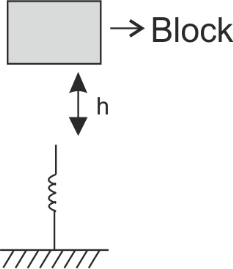
Then there will be some velocity so we will need to calculate the velocity of the block falling from a height of
We have a gravitational force which is acting downward direction and height of the block is
So,
Here, initial velocity is zero, so it will become
Velocity of block falling from height of
When the block will fall in spring then spring will compress and velocity becomes zero.
Means kinetic energy is converted into spring potential energy
So, we can write that,
Kinetic energy is equal to spring potential energy.
We know that, kinetic energy formula is
And spring potential energy formula is
Putting this value in equation
Maximum compression of spring is
Note :
(a) Velocity is a ratio of displacement to change in time. Distance and displacement are the main key point of velocity. There will be no velocity if there is no displacement in the object or if the object does not cover any distance.
There are two kinds of velocity
(i) initial velocity: velocity of body at beginning of time given is called initial velocity.
(ii) Final velocity: velocity of body at the end of time given is called final velocity.
Mass is the amount of matter in the body. Mass does not change with time. It only changes in extreme cases like a huge amount of energy is given or taken from a body. Mass and weight are different things in physics SI unit of mass in kilogram (kg)
Read questions properly and remember the formulas and steps of solution.
You can directly calculate answer by using this formula
Where,
Where,
Where,
Complete Step By Step Answer:
Given,
Let's imagine a spring is there and a block

Then there will be some velocity so we will need to calculate the velocity of the block falling from a height of
We have a gravitational force which is acting downward direction and height of the block is
So,
Here, initial velocity is zero, so it will become
Velocity of block falling from height of
When the block will fall in spring then spring will compress and velocity becomes zero.
Means kinetic energy is converted into spring potential energy
So, we can write that,
Kinetic energy is equal to spring potential energy.
We know that, kinetic energy formula is
And spring potential energy formula is
Putting this value in equation
Maximum compression of spring is
Note :
(a) Velocity is a ratio of displacement to change in time. Distance and displacement are the main key point of velocity. There will be no velocity if there is no displacement in the object or if the object does not cover any distance.
There are two kinds of velocity
(i) initial velocity: velocity of body at beginning of time given is called initial velocity.
(ii) Final velocity: velocity of body at the end of time given is called final velocity.
Mass is the amount of matter in the body. Mass does not change with time. It only changes in extreme cases like a huge amount of energy is given or taken from a body. Mass and weight are different things in physics SI unit of mass in kilogram (kg)
Read questions properly and remember the formulas and steps of solution.
You can directly calculate answer by using this formula
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




