
A ball falls from a height such that it strikes the floor of lift at 10 m/s. If lift is moving in the upward direction with a velocity 1 m/s, then velocity with which the ball rebounds after elastic collision will be
A. 11 m/s
B. 10 m/s
C. 12 m/s
D. 9 m/s
Answer
501.6k+ views
2 likes
Hint: Two particles are moving in the same direction in a straight line with velocities
After collision velocity of the first particle and second particle are
Coefficient of restitution is the ratio of relative velocity of separation after collision to relative velocity of approach before collision.
Coefficient of restitution,
For, elastic collision,
Complete step by step answer:
Let us consider ball as particle 1 and lift as particle 2.
See the diagram before collision:
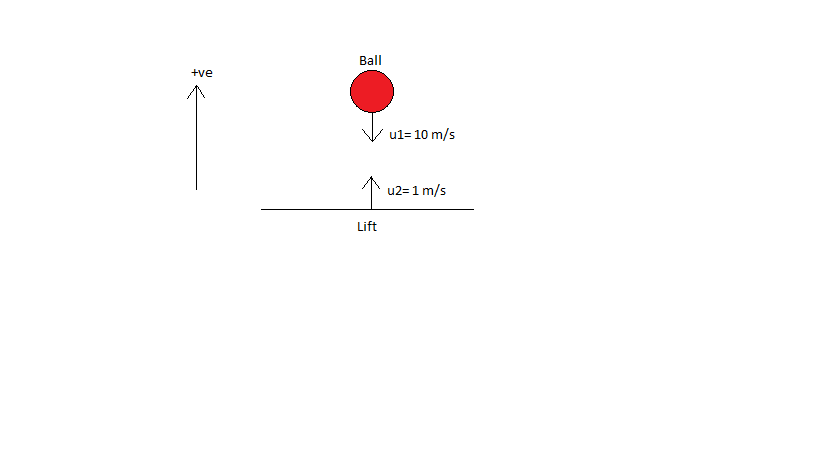
Taking upward direction as positive,
Velocity of ball before collision,
Velocity of lift before collision,
Now, we should consider the situation after the collision.
After collision lift will continue it’s motion 1m/s upwards and the ball will rebound with a velocity, say
So, Velocity of lift after collision,
Velocity of ball after collision be
As it is an elastic collision, then
We know,
Now, putting the values,
So, Ball rebounds with 12m/s velocity upwards after elastic collision.
So, the correct answer is “Option C”.
Note:
We can take
After collision velocity of the first particle and second particle are
Coefficient of restitution is the ratio of relative velocity of separation after collision to relative velocity of approach before collision.
Coefficient of restitution,
For, elastic collision,
Complete step by step answer:
Let us consider ball as particle 1 and lift as particle 2.
See the diagram before collision:

Taking upward direction as positive,
Velocity of ball before collision,
Velocity of lift before collision,
Now, we should consider the situation after the collision.
After collision lift will continue it’s motion 1m/s upwards and the ball will rebound with a velocity, say
So, Velocity of lift after collision,
Velocity of ball after collision be
As it is an elastic collision, then
We know,
Now, putting the values,
So, Ball rebounds with 12m/s velocity upwards after elastic collision.
So, the correct answer is “Option C”.
Note:
We can take
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE




